Antiderivative calculations play an important role in different branches of mathematics, sciences, and engineering fields. Understanding antiderivatives is crucial for students to solve mathematical problems, especially for solving the problem of calculus.
With the help of antiderivative solves many mathematical problems using its different rules and techniques. Moreover, it helps us find the solution to the initial value problem that plays an important role in our practical life.
Many students are worried about finding the antiderivative of the different functions. Now, you don’t worry about that, here we provide a complete guide to solving different problems and providing detailed information about the Antiderivative, its rules, and techniques for finding it.
What is an Antiderivative?
Antiderivative of a function is determined of those functions whose derivatives are taken. In other words, the Antiderivative of a function becomes again a function that is determined by the “F(x)” if its derivative function is already taken that represented by “f(x)”.
Antiderivative is also known as the reverse process of differentiation or derivative. If differentiation is about determining the function’s rate of change with respect to the specific variable, then antiderivative is finding the original quantity from its rate of change.
The process of finding the antiderivative of the different functions is known as the “integration” and the sign used is known as the “integral”, the integral of the function is represented as “∫f(x) dx”. Due to the representation of integral or bounds, there are exit types of integral.
Types of integral
There are two types of integral: Definite Integral & Indefinite Integral.
Definite integral mathematically can be represented as,
∫ f(x) = F(x) + c
While, indefinite integral mathematically can be written as below and solved with the help of the fundamental rule of calculus,
∫ab f(x) = F(b) – F(a)
It plays a fundamental role in calculus because it helps to solve the initial value problem, rectilinear motion problem, and shows a graphical representation of the function as the area under the curve.
Antiderivative Rules
Some basic rules are used to find the antiderivative of sums, differences, and products of the different functions. Let f(x) & g(x) be two functions. Here, we explain its rule with its formula.
Sum rule
The antiderivative of the sum of two functions is simply the antiderivative of the individual function. The sum rule can be represented as:
∫ [f(x) + g(x)] dx = ∫f(x) dx + ∫g(x) dx = F(x) + G(x) + c
Difference rule
The antiderivative of the difference of two functions is equal to the difference of the antiderivative of individual functions. It can be written as:
∫ [f(x) – g(x)] dx = ∫f(x) dx – ∫g(x) dx = F(x) – G(x) + c
Power rule
It is an important rule to determine the antiderivative, if the product of the function & its derivative without power such as “f(x) = xn” where “n” is the real number except “-1”. Its mathematical formula can be represented as,
∫ fn(x). f’(x) dx = f n+1(x)/(n+1) + c
simply:
∫ xn dx = xn+1/(n+1) + c
Constant rule
The antiderivative of the constant of the function is simply the variable of those derivatives taken. Its mathematical form can be written as:
∫ n dx = n ∫dx = n x + c
Techniques for finding Antiderivative
The above rules help to find the antiderivative of the sum, difference, and power of the simple functions. But not determine the solution of the product of the two different functions and some complicated functions that are the combination of the two trigonometric functions and the composition of two functions.
For this use the below techniques to determine the solution of the function.
- Substitution: This technique is useful if the function is given in the form of composite functions such as: f(x) = (3x2 + 1)5.
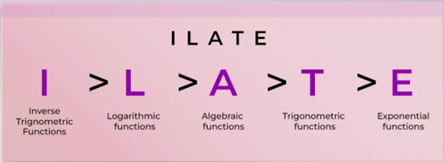
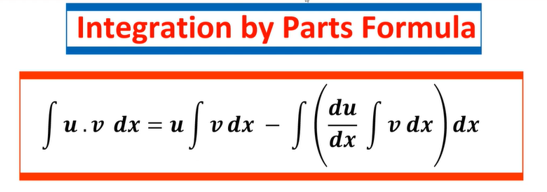
- Integration By Parts: It helps to find the integration of the product of the two functions with the help of the term “ILATE” which decides the first and second functions.
Some Special Antiderivative Formulas
Some useful trigonometric and exponential formulas are described in the following table.
| Functions | Formula |
| Cos(x) | ∫ [cos(x)] dx = sin (x) + c |
| Sin (x) | ∫ [sin(x)] dx = -cos (x) + c |
| Tan(x) | ∫ [tan(x)] dx = -ln(cos(x)) + c |
| Sin-1(x) | ∫ dx/ (b2 – x2) ½ = [ sin -1(x/b)] + c |
| Cos-1(x) | ∫-dx/ (b2 – x2)1/2 = [ cos-1(x/b)] + c |
| Tan-1(x) | ∫ dx/ (b2 + x2) = 1/b [ tan-1(x/b)] + c |
| Exponential | ∫ enx dx = enx / n + c Where “c” is a constant. |
| Reciprocal | ∫ f’(x)/(f(x))dx = ln f(x) + c or ∫ 1/xdx = ln(x) + c |
How To Evaluate the Antiderivative of the Function
To evaluate the antiderivative/integration of the function follow the below steps.
- First, let the value is equal to the function according to the independent variable such as x,y, & z then its function: f(x), f(y), & f(z) respectively.
- Now, apply the integral on both sides with respect to an independent variable.
- Now, apply the suitable rule according to the situation such as: the sum rule & difference rule.
- Finally, solve the integral using the formulas of integral or techniques of integral and get your final result after simplification.
Examples of antiderivative
Here, we provide some examples for a better understanding of the use of the rules of antiderivative and how to apply the different techniques of antiderivative according to the situation.
Example 1: Find the antiderivative if the function is “x2 (x – 1)”.
Solution
Step 1: Let the given value is equal to f(x) according to an independent variable.
f(x) = x2 (x – 1) = x3 – x2
Step 2: Apply integral on both sides w.r.t the “x”
∫ f(x) dx = ∫ (x3 – x2) dx
Step 3: Apply the Difference rule and solve the integral using antiderivative formulas.
∫ f(x) dx = ∫ x3 dx – ∫ x2 dx
Using the power rule formula then we get a final result.
∫ f(x) dx = x4 / 4 – x3/3 + C
Alternatively, to remove the difficulty of long manual calculations use an Antiderivative calculator to make your calculations easy and faster with detailed steps.
Example 2: Find the antiderivative of 3 Sin(x) + 4 Cos(x) using integral formulas.
Solution:
Step 1: let the given value be equal to a function “f(x)” with respect to its independent variable.
f(x) = 3 Sin(x) + 4 cos(x)
Step 2: Apply the integral on both sides w.r.t to the independent variable “x”.
∫ f(x) dx = ∫ [3 Sin(x) + 4 Cos(x)]
Step 3: Separate the integral with the help of the sum rule and solve the integral.
∫ f(x) dx = ∫ [ 3 Sin(x)] dx + ∫ [4 Cos(x)] dx
= 3∫ Sin(x) dx + 4 ∫ 4 Cos(x) dx
Apply the integral formula of “Sin(x)” & “Cos(x)”.
= 3[ -(cos(x)] + 4 [ sin (x)] + c
Thus, ∫ f(x) dx = – 3 cos(x) + 4 sin (x) + c
Application of Antiderivative
Antiderivative plays an important role in various fields of mathematics and sciences such as physics, engineering, computer science, statistics, and geometry.
Physics & Engineering
In physics, antiderivative helps to find the solution of the rectilinear motion problem and initial value problems. It helps to find the position function & acceleration function from the velocity function which changes with respect to the time.
In engineering, helps to find the work done by a variable force with its displacement by determining the integration of the force function.
Statistics
In statistics, Antiderivative helps to find the cumulative distribution function from the probability density function.
Computer Science
In computer science, some algorithms particularly those involving optimization and analysis of changes use antiderivative or some special function to determine the solution.
Geometry
Antiderivative plays an exclusive role in geometry to determine the area under the curves and the volume of the solids by integrating the cross-sectional area along its axis such as: x-axis, and y-axis.
These applications determined that antiderivative is not just a theoretical construct but also has practical uses in solving real-world problems. That shows how much antiderivatives are necessary for our practical life and how help us in different disciplines.
Final words
In this comprehensive guide, you gained knowledge of antiderivative calculations with detailed concepts that help the student to find the Antiderivative of different problems. We explored the concept of antiderivatives and their different integral types. Moreover, mastered essential antiderivative rules and techniques. We’ve also explored practical applications that showed how antiderivatives play a crucial role in various fields like physics, engineering, and even computer science. Finally, for a better understanding of antiderivative, we solved different examples with detailed steps. I hope by reading this guide you can solve your related problem easily.




